Pizza and Panini

PIZZA AND PANINI

This piece is in collaboration with Prof. Krishnan Shankar, Professor of Mathematics at the University of Oklahoma. 

The Oracle Asks 

The Sanskrit grammarian Panini is at his friend Socrates’ place in Athens.

-+- 

Boy. Here is the tea.

Socrates. Thank you.

Panini. The boy, he understands Greek Mathematics, does he not?



Socrates. Yes, indeed; he was born in the house.



Panini. Can you talk to him about mathematics?

Soc. Certainly. Attend now to the questions which I ask him, and observe whether he learns of me or only remembers.

 

Pan. I will.



Soc. Tell me, boy. Do you know that a figure like this is a triangle?

Boy. I do.

Panini. You see that part ABC? It looks like a slice of pizza.

Boy. You I like!



Soc. And you know that an isosceles triangle figure like this has these two lines equal?

Pan. An isosceles triangle is a triangle which has two sides that are equal. The third side of the triangle may have a different length.

Boy. Certainly. I understood Socrates.

Soc. And you know that a triangle figure with these two lines equal has the third line equal to sqrt(2) times the either of the first two lines?



Pan. Basically, if you have a triangle with two equal sides R and S that are at right angles to each other, then the third side will have a length that is sqrt(2) times the length of R.

Boy. Without a doubt.

Soc. And this arc which I have drawn with A as center creates a line AC that is also equal?



Boy. Yes.



Soc. A triangle may be of any size?



Boy. Certainly.

Soc. It could be smaller than this teacup, or larger than this house?

Boy. Yes.


(Anand (observing from Chandrayaan)) [Aside].  Let us see where he is going with this.


Soc. And if one line of the triangle be of integer feet, and the other line cutting into two parts be of integer feet, what will the difference be? Let me explain: if in one direction, the line was of integer feet, and in other direction, the line is also of integer, will the difference be of integer feet as well?

(Ravi (observing from Chandrayaan)) [Aside]. Few people would understand what Socrates said right there. If you didn’t understand it, please move along. It is not necessary for you to understand that bit.

Boy. I did not understand that.

Panini. I did understand you. You are saying that if two sides of a figure have lengths that are exact integers, then will the difference between the two lengths also be an integer? The answer to that is “Yes”. Please continue. 




Soc. But since this side is also of integer feet, there are two sides of integer feet?


Pan. There are. 



Soc. And the difference of two integer feet is also an integer foot? 



Pan. Yes.

(Ravi [Aside]) The boy is completely lost. He seems to be wishing he were somewhere else.

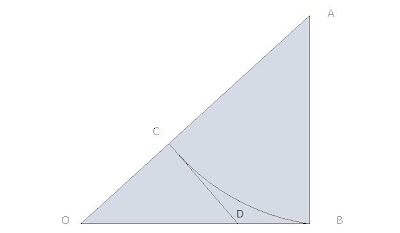
Panini. Let me prove now why sqrt(2) is irrational. An irrational number is a number that cannot be expressed in the form x/y where x and y are both integers. Now, take this figure. It is in the shape of a triangle. Now, if sqrt(2) were rational, we could construct a right isosceles triangle with all integer sides. Let ABO be the smallest such triangle. But if we could construct one (call it ABO), then we could also construct a smaller one, CDO. It would be like slicing the pizza slice into two. Point C is obtained by drawing an arc of a circle with center A. Point D is obtained by drawing a perpendicular at C. Why must CDO also have integer sides? Because first, AC = AB. Since AC and AO are integers, OC must also be an integer. Now, CD = OC because CDO is a right triangle that is isosceles. But then, C and B are both the tangent points to the circle from the point D, and so CD must equal DB. This means that OC, CD and OD are all integers. And we can do this ad infinitum. But that is a contradiction because you cannot construct triangles that are arbitrarily small, and still have integer sides. Another way to put it is that this contradicts our assumption that ABO is the smallest right isosceles triangle with all integer sides. QED.

Soc. Nice! And may be you could turn this into a shloka. But does a shloka or a poem count as proof?

Panini. Sure, it does. And it is twice as elegant as prose. And now I have to go. Thanks for the tea.

Soc. You are welcome. Thanks for the sheet of that pliable material that you call paper. It has some very interesting things written on it. I have never seen anything like it. It is from the future, you tell me. The things that people are going to be able to do in the future with things like this. It will be an age of innovation. Just imagine! And so, where are you off to?

Panini. To Brahmagupta’s house. What a brilliant man of mathematics he is. A man who may one day be adjudged perfect in this species of wisdom.

(Exit Boy)

(Anand [Aside]) This was written in the style of one of Plato’s works. So it might seem a bit dense. If you understand everything up to the point where I first come in, you are all set. Now, back to Athens.

-+- 

And Panini leaves knowing a little more than he did before about Greek Mathematicians. There is one thing Panini does not understand, though. Why on earth is Socrates so long winded and why can’t Greek mathematicians, in general, be more to the point?

Update (June 1): I have posted a short comment on this piece on this blog (Linked here).